ELECTROCHEMICAL CAPACITORS
Their Nature, Function, and Applications

Historical introduction

A great deal of scientific and technological research has been reported in the scientific literature since about 1990. An extensive and detailed account of this has been given in the author's monograph on "Electrochemical Supercapacitors: Scientific Fundamentals and Technological Applications" (1999).

Scientific introduction

The nature of electricity took a long time to be understood, from the early experiments on electrostatic electricity in the mid-18^th century, for example by Galvani, through the time of the invention of the first electric battery by Alessandro Volta (Volta's "Pile") in 1800, on to Faradays's and Davy's monumental discoveries on the chemical origin of electricity generated by Volta's pile. At first, two "kinds" of electricity were postulated: "animal electricity", as in the works of Galvani on stimulation of the frog's leg nerve by contact between two dissimilar metals and later, "Voltaic electricity" generated chemically from a Volta pile of zinc and silver or copper plates separated by paper wetted with an acid or salt solution (Conway, 2000).

In parallel with these discoveries were extensive works on electrostatic electricity generated for example by the rubbing of naturally occurring amber or by the so-called Wimshurst machine (a rotating circular plate, containing insets of amber-like material, rubbing against charge-collector plates connected overall to a Leyden Jar or a spark-gap). It was from this direction of research on electricity that the invention of the electric condenser arose, referred to as the "Leyden Jar", and capable of storing electric charge generated by a Wimshurst machine. Such a jar had the "capacity", depending on its dimensions and materials of construction, of storing electric charge by bringing it together in a condensed way (hence the term "condenser") on the surfaces of a Leyden Jar at a certain two-dimensional charge density.

The principle of design and operation of the Leyden Jar and all subsequent regular condensers or capacitor devices, is as follows. Two metal surfaces that constitute electrodes are separated at some small distance either in air (or vacuum) or on each side of a liquid or solid film, referred to as the "dielectric", a term first used by Michael Faraday . For a given separation of the electrode plates, the capacitance developed per unit area of the two plates depends on the properties of the dielectric between the plates characterized by its so-called dielectric constant.

Fig. 1. Leyden Jar, the first capacitor or "condenser".

In the case of the Leyden Jar (Figure 1), the material (glass) of the jar itself serves a the dielectric medium and the contact plates were metal foils wrapped, inside and out, around the cylindrical surfaces of the jar. The electrical contact to the inner surface foil was by means of a conducting electrolyte solution (or originally by ordinary water itself) in which was immersed a conducting metal electrode for electrical contact. The device was charged by joining two wires from the inside electrode and the outside foil to an electrostatic machine of the Wimshurst type. In later experimentation, the Leyden Jar capacitor was connected to the electrodes of a Volta's pile or battery for charging. This was the first-generation capacitor for storage of electric charge.

The nature of electric charge remained elusive until much later (1897) when J.J. Thomson identified and characterized the fundamental entity of electric charge as the "electron", present ubiquitously in all atoms of the Universe and identified, in his experiments, by means of experiments on gases at low pressures in gas-discharge tubes (Crookes tubes or neon lights). The electron charge was determined independently by Townsend and by Millikan (Glasstone, 1940), and was shown to be equivalent to Faraday's constant for the relation between extent of passage of charge and extent of chemical change (as related by Faraday's Laws) caused by electrolysis of conducting solutions, when calculated on a "per gram-atom" or "gram-equivalent" basis.
 

Relation of capacitance to geometry and dielectric constant of a capacitor

Electrochemical capacitors are a special kind of capacitor based on charging and discharging the interfaces of high specific-area materials such as porous carbon materials or porous oxides of some metals. They can store electric charge and corresponding energy at high densities in an highly reversible way, as does a regular capacitor, and hence can be operated at specific power densities (in watts/kg) substantially higher than can most batteries. Their capacitance for a given size of the device is thus much higher, by a factor of 10,000 or so, than those achievable with regular capacitors. For this reason proprietary names such as "Supercapacitors" or "Ultracapacitors" have been coined to describe their performance.

While they function formally like rechargeable batteries in storing or delivering electric charge, their mechanisms of charge storage are quite different, in most cases, from those operating in batteries. Thus, electrochemical capacitors are not substitutes for batteries but rather are to be regarded as complementary to them for charge storage or delivery. They can offer advantageously fast charging or discharging rates over most batteries of comparable volume but their energy density is usually less, by a factor of 3 to 4, than that of batteries. Their high power or power densities, however, enables them to be employed in interesting complementary ways in hybrid systems with batteries.

An important difference between charging a capacitor and charging a battery is that there is always an intrinsic increase of voltage on charge (or decrease on discharge) of a capacitor as the charge per cm² is increased or decreased. In contrast, an ideal battery has a constant voltage during discharge or recharge except as the state of charge approaches 0 or 100%. Although practical batteries exhibit some dependence of cell voltage on state of charge, especially lithium-intercalation batteries, the latter for fundamental reasons arising from intercalation. (See the Appendix for further details.)

The double-layer capacitance at electrode interfaces

Nature of electrical double layers

Fig. 2. Models of the double layer as historically developed: a) Helmholtz model b) Gouy-Chapman model of the diffuse layer c) Stern's model, combining (a) and (b) d) Grahame's later model and e) Model of Bockris, Devanathan and Muller showing presence and orientation of solvent dipoles.

Helmholtz envisaged a capacitor-like separation of anionic and cationic charges across the interface of colloidal particles with an electrolyte. For electrode interfaces with an electrolyte solution, this concept was extended to model the separation of "electronic" charges residing at the metal electrode surfaces (manifested as an excess of negative charge densities under negative polarization with respect to the electrolyte solution or as a deficiency of electron charge density under positive polarization), depending in each case, on the corresponding potential difference between the electrode and the solution boundary at the electrode. For zero net charge, the corresponding potential is referred to as the "potential of zero charge".

In response to positive or negative electric polarization of the electrode relative accumulations of cations or anions develop, respectively, at the solution side of the charged electrode. If, for energetic reasons, the ions of the electrolyte are not faradaically dischargeable (that is no electron transfer can occur across the interface ("ideally polarizable electrode", for example a mercury electrode, Grahame 1947 and Parsons 1954), then an electrostatic electrical equilibrium is established at the interface resulting in a "double layer" of separated charges (electrons or electron deficiency at the metal side and cations or anions at the solution side of the interface boundary), negative and positive, across the interface.

The difference of potential extends beyond the immediate layer of solvated ions in the compact, capacitor-like (Helmholtz) region, out into solution, so that a further diffuse region capacitance (the diffuse-layer capacitance "C_diff") arises. It combines with that of Helmholtz's region "C_H" in series. (See the Appendix for further details.)
 

The Helmholtz region capacitance "C_H" is of special significance for electrochemical capacitors since it is directly dependent on accessible electrode area and has large values (relative to those for regular dielectric capacitors) between about 16 µF/cm² and about 40-50 µF/cm², depending on electrode potential, the chemical nature of the metal surface, chemical nature of the solvent, and the types of ions (and their solvation by the solvent) present in the electrolyte solution.

The most extensively studied metal surface, with respect to its double-layer capacitance, is that of mercury in various solvent media, especially water.

It was mentioned that the specific capacitances of electrode double layers are very large, some 10,000 times those of ordinary dielectric capacitors, per /cm² area. The reason for this is that the separation of charges in electrochemical double layers is on the order of 0.3-0.5 nm instead of 10 to 100 nm with oxide-film dielectrics (electrolytic capacitors) or 1000 nm with very thin mica or polystyrene dielectric-film hardware capacitors.

Hence, it is seen that with large specific-area porous electrodes, for example at carbons having say 1000 m²/g of material and exhibiting, say, 15 µF/(real cm² of double-layer capacitance in some suitable electrolyte solution, the accessible capacitance "C" is 1000 (m²/g) × 10,000 (cm²/m²) × 15 (µF/cm²) = 150 million µF/g, that is 150 farads/g, a very large capacitance! Hence the term "supercapacitors" or "ultracapacitors" for devices based on double-layer capacitance at high-area substrates.

The high degree of reversibility of charge acceptance and delivery, and hence capability for excellent operating power levels compared with batteries of comparable size arises because no slow chemical processes or phase changes take place between charge and discharge as they do in most battery-type electrical charge generating devices.

It is the essence of battery-type charge/discharge processes that faradaic processes take place leading to major chemical and structural changes of the electrochemical reactive materials, for example conversions of lead dioxide to lead sulfate and lead metal to lead sulfate in discharge of the lead-acid battery which, as is well known, limits charge/discharge to a cycle life of 1000 to 3000, depending on rates of charge and discharge, and temperature. By contrast, electrochemical double-layer and oxide-type (see below) capacitors can exhibit cycle lives up to one million under suitable conditions. This is because, ideally at least, only storage and delivery of electrostatic charge takes place at the extended two-dimensional interface of high-area materials and no irreversible or slow chemical phase changes take place as they do between three-dimensional chemical materials in rechargeable batteries. This is a fundamental difference between the electrochemical behavior and properties of electrochemical capacitors relative to those of batteries.

Electrical irreversibility in electrochemical capacitor charging and discharge: rate effects

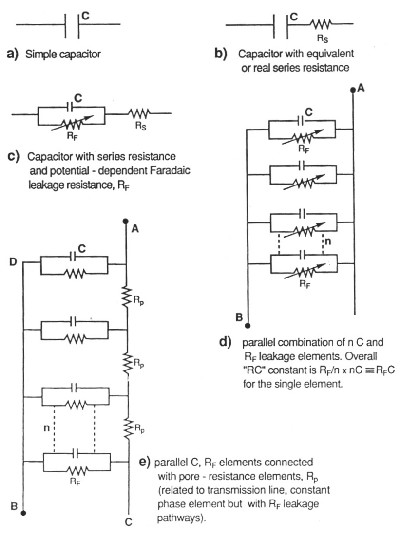Fig. 3. Hierarchy of equivalent circuits for capacitors and electrochemical capacitors showing "transmission-line" circuit for the latter, in the case of a porous carbon electrode.

In the simplest analysis, any practical capacitor device behaves as if an ohmic resistance is in series with it, the so-called equivalent (or real) series resistance (Figure 3).

The presence of real or equivalent series resistance in the operating equivalent circuit of any capacitor introduces an ir potential drop in the process of charging or discharging and this drop depends, of course, on the charging rate (current) leading to distortion of the charging curve of accumulated charge against voltage, in time. When the distributed resistance effect also operates, as it normally does, the distortion effect becomes more complex but has been experimentally and computationally evaluated (de Levie, 1963).

The above effect causes limitation of rates at which the capacitor can be charged or discharged and, for ac modulated charging, it also introduces a frequency-dependent phase angle (normally -90^o) between the modulated applied voltage and the resultant charging current. This also applies to other, non-constant charging modes.
 

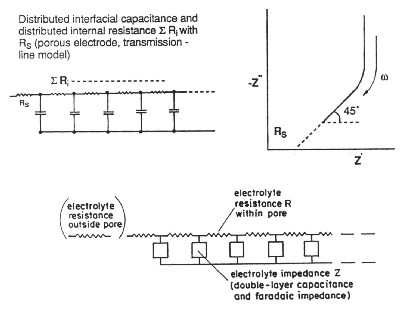Fig. 4. de Levie transmission-line model for resistance/capacitance matrix in an high-area porous electrode.

In practice, with a porous electrode, the situation is much more complex since the matrix of microscopic pores within the macroscopic electrode structure offers a complex, series/parallel equivalent circuit comprised of a distribution of ohmic (resistive) and capacitive elements which leads to the whole electrode having a non-uniform effective resistance and capacitance, dependent on frequency (in ac modulation) or on the time-scale of pulsed or non-constant rates of charging. As shown in the classical work of de Levie (1963), the equivalent circuit for such electrodes is that of a transmission line (Figure 4) having a -45^o phase angle (Figure 5) characterizing its impedance behavior.
 

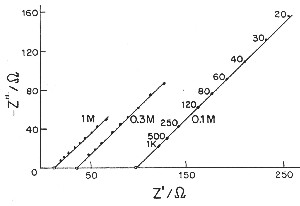Fig. 5. Complex-plane impedance plots (imaginary capacitive component against real ohmic component) for a porous electrode according to de Levie.

This situation leads to limitation of the rates of which charging or discharging of porous capacitor electrodes can be conducted and is additional to any equivalent series resistance effects a practical cell may experience due to cell design and electrolyte resistance.

de Levie has pointed out that the distributed R/C effect in porous electrodes is equivalent to restrictions of power due to ohmic ir drop (that is the potential drop due to current passing through resistive elements in the matrix). Thus there is a "penetration effect" into the electrode matrix due to attenuation of for example a modulation or time-dependent transient charging voltage so that not all of the depth or width, or diameter of the electrode structure is subject to a uniform charging rate. This introduces a non-chemical irreversibility in the charge/discharge behavior of the electrochemical capacitor device which is electrically demonstrable.

It must be stated, however, that in state-of-the-art developments of electrochemical capacitors, using aqueous-solution electrolytes, the above distributed-resistance effect has been substantially minimized so that devices having high operating power have been successfully engineered and marketed. Nevertheless, with non-aqueous electrolyte capacitors, which have higher operating voltages up to 3.0 to 3.5 V (hence 9 to 12 times energy density which depends on the square of maximum operating voltage), the distributed ohmic effects are more significant so that achievable operating power levels are less than those attainable with aqueous electrolyte devices.
 

Electrochemical capacitors based on pseudocapacitance

However, when the process is surface limited, and is proceeding in several one-electron stages, a broad range of significant capacitance values arises as is found with ruthenium dioxide electrodes where the pseudocapacitance is almost constant (within 5%) over the full operating voltage range. Some other metal oxides behave similarly but only over smaller operating voltage ranges. The ruthenium dioxide pseudocapacitance provides one of the best examples of electrochemical (pseudo)capacitance as, in addition to the almost constant capacitance over a wide voltage range, its reversibility is excellent, with a cycle life over several hundred-thousand cycles. Furthermore, the pseudocapacitance can increase the capacitance of an electrochemical capacitor by as much as an order of magnitude over that of the double-layer capacitance. However, its cost prevents its large-scale use so that it has been employed mainly in military applications.

Another type of material exhibiting pseudocapacitive behavior that is highly reversible is the family of conducting polymers such as polyaniline or derivatives of polythiophene. These are cheaper than ruthenium dioxide but are less stable, giving only thousands of cycles (still quite attractive) over a wide voltage range. (See the Appendix for a more detailed discussion of the pseudocapacitance.)

Applications and technology

Fabrication of electrochemical capacitors

Hybrid systems

Another type of hybrid system is where ruthenium dioxide is used as a second oxide component, acting as a pseudocapacitance in electrolytic capacitors (the Evans Hybrid Capacitor). This gives indirectly, an improved capacitance density for the overall two-electrode device.

Another interesting hybrid type, currently under investigation in our own and other laboratories, is a combination of a double-layer carbon (electrochemical capacitor) electrode combined to work against a rechargeable battery electrode, for example a lead-acid positive plate (acidic solution) or a nickel battery positive plate (alkaline solution). This type of device (called an asymmetric capacitor) enables almost all of the charge residing on the capacitor-type electrode to be utilized on discharge in contrast to the battery-type electrode while, with a symmetrical capacitor made with two similar double-layer capacitance electrodes, each electrode is only half discharged from its initial voltage, relative to the other electrode, when the cell discharge voltage reaches zero, therefore delivering less electrical energy than the hybrid.

Another application is in electrical research experiments where very high energy and high-rate discharges are required, for example through gases for high-energy spark or arc generation.

A variety of other applications has been envisaged in the literature (Conway, 1999). Examples are: cold-start assist for diesel locomotives; emergency back-up power for computer systems; stationary power-system load leveling or bridging for short-period power outages; energy source for initial heating of catalytic converter units; energy collection and storage from windmill dynamos.

For society at large, the use of capacitor/battery hybrid systems for improvement of electric-vehicle (EV) performance will assist the adoption of EV transportation systems with zero or diminished nitrogen oxide and carbon dioxide atmospheric contamination. However, such a transition to an "EV lifestyle" will probably not become substantial for another one to two decades.

Appendix

Relation of capacitance to geometry and dielectric constant of a capacitor

[1] C = A ε / 4 π d

or, in terms of so-called rationalized units,

[2] C = Aε ε_o / d

where ε_o (= 8.84 × 10^-12 farads/m) is the dielectric permittivity of free space. The units of "C" are in farads or coulombs per volt, that is coulombs stored by the capacitor per volt across its two electrodes.

Some important differences between capacitors and batteries

[3] C = q/V or q = CV

In contrast, an ideal battery has a constant voltage during discharge or recharge except as the state of charge approaches 0 or 100%. (Practically, most batteries exhibit some dependence of cell voltage on state of charge, especially lithium-intercalation batteries, the latter for fundamental reasons arising from intercalation).

The consequence of the above difference, based on Equation [3], is that the energy stored by a capacitor is 1/2 CV² or 1/2 qV while, for a battery, the corresponding stored energy (or energy density) is qΔV, twice as much as that for a capacitor charged to the same cell voltage "ΔV". Thus, the stored energy in a capacitor device increases as the square of the cell voltage "V" as charge is accumulated. This is an important difference between capacitor and battery cell behavior and affects the interfacing between such systems in hybrid devices.

It was noted above that the energy stored in a capacitor cell, charged to a voltage "V" is 1/2 CV² and "V" increases as charge "q" accumulates on its plates determined by "C". The charging energy 1/2 CV² arises in the following way. For a capacitor being charged from an initial voltage V = 0 to a final value "V_f" the energy "E" stored will be a free-energy "G" (charge times voltage):

[4]

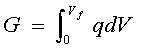
At any state of charge, q = CV (Equation [3]), so that

[5]

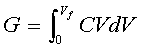
[6] G = 1/2CV_f²

Double-layer capacitance

[7] C_dl = dq/d(ΔΦ) or Δq/ΔΦ

The difference of potential extends beyond the immediate layer of solvated ions in the compact, capacitor-like (Helmholtz) region, out into solution, so that a further diffuse-layer capacitance "C_diff" arises. It combines with the capacitance of Helmholtz region "C_H" in series, electrically, so that

[8]

Pseudocapacitance

This kind of pseudocapacitance can originate when an electrochemical charge-transfer process takes place to an extent limited by a finite quantity of reagent or of available surface, the latter in the case of adsorption (for example of hydrogen), with charge transfer. Several examples of pseudocapacitance can arise as follows, but the "C_Φ" function is usually not constant and, in fact, is usually appreciably dependent on potential or state of charge.

a) When an electrochemical adsorption process, arising from charge transfer, takes place at an electrode surface, for example in the process of electrosorption of H atoms discharged at Pt electrode surfaces in aqueous acid solution by the process:

[9] H₃O⁺ + Pt + e^- ==> H₂O + Pt/H

so-called underpotential deposition since the above reaction takes place over a potential range "ΔV" of about 0.35 V positive to the equilibrium potential for H₂ gas evolution in the electrolysis of water.

Fractional H coverages "Θ" at Pt can increase continuously, over the above electrode potential range, from 0 to 1, prior to evolution of H₂ and each atom of H deposited requires passage of one electron of electric charge. Hence, a pseudocapacitance "C_Φ" arises from the relation between charge passed "q" and "ΔV", and "q" is faradaically related to the coverage fraction "Θ" of electrodeposited H. A full monolayer (Θ ==> 1) of H requires passage of about 210 µC of charge "q₁" per cm² of a smooth Pt surface.

[10] C_Φ = q₁ dΘ/dΔV

"Θ" is related to electrode potential "V" by the relation

[11] Θ/(1 - Θ) = K exp (VF/RT)

b) A number of electrochemical reactions involving species in solution, such as Fe⁺⁺⁺/Fe⁺⁺ or [Fe(CN)₆]^3-/[Fe(CN)₆]^4-, known as redox processes, take place at inert electrodes, for example Au or Pt, with a logarithmic relation (the Nernst equation) between the extents of oxidation/reduction between the redox couple pair of ions. Thus, for example

[12] E = E^o + (RT/F) ln a[Fe(CN)₆]^3-/a[Fe(CN)₆]^4-

where "a" represents the respective activities of the two ions of the redox couple. The above Equation [11] can be rearranged in terms of the fractions of a given chemical quantity of the redox ions in the reversible reaction

[13] [Fe(CN)₆]^3- + e^- <==> [Fe(CN)₆]^4-

that exist in the course of reduction (or reoxidation) as a function of the potential "E" in relation to its so-called standard value "E^o" in Equation [12]. E = E^o when the reagent ion activities (concentrations) are equal so that the ln function is zero. The rearranged form of Equation [11] is in terms of the molar quantities "Q_ox" and "Q_red" in relation to the total reagents Q_ox + Q_red (= Q) and reads

[14]

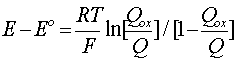
with Q_red/Q = 1 - Q_ox/Q. Then it is seen that Equation [14] has the same form as that of Equation [11] (after taking logarithms) for electrosorption and its derivative is a capacitance quantity.

c) A similar logarithmic relation in X / (1 - X) applies to absorption of Li⁺ ions into Li-intercalation hosts in Li-ion battery electrochemistry where "X" is the fractional occupancy of available intercalation sites in the intercalation host material, for example TiS₂, CoO₂, etc. Hence, Li⁺ ion intercalation formally exhibits a pseudocapacitance, though the Li⁺ ion systems are normally referred to as battery devices.

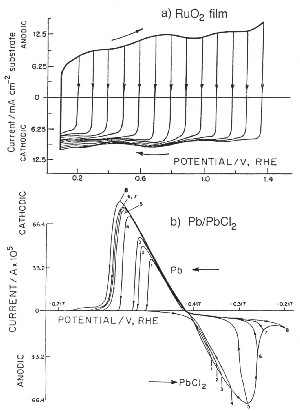Fig. 6. Comparison of cyclic voltammetry behavior of a reversibly chargeable electrochemical capacitor material (RuO2) and a battery-type (Pb/PbCl2) like in the lead-acid battery.

In each of the three types of pseudocapacitance systems, "C_Φ" is substantially dependent on potential, having a large maximum of about 2200 µF/cm² at "Θ", "Q_ox/Q", or "X" equal to 0.5, but with appreciable values of "C_Φ" arising only over a potential range of about 120 mV which is too narrow to be of value for practical applications.

However, when the redox process is a surface one, proceeding in several one electron stages, a much broader range (1.4 V) of significant "C_Φ" values arises as is found with RuO₂ electrodes where "C_Φ" is almost constant within 5% over that voltage range. Some other transition-metal oxides behave similarly but only over smaller operating voltage ranges, about 0.6 to 0.8 V (Conway, 1997).

The case of RuO₂ pseudocapacitance provides one of the best examples of electrochemical (pseudo)capacitance as its "C_Φ" is almost constant over a 1.4 V voltage (Figure 6) range and its reversibility is excellent, with a cycle life over several hundred-thousand cycles. However, its cost prevents its large-scale use so that it has been employed mainly in military applications.

Another type of material exhibiting quasi-redox behavior that is highly reversible is the family of conducting polymers such as polyaniline or derivatives of polythiophene. These are cheaper than ruthenium dioxide but are less stable, giving only thousands of cycles (still quite attractive) over a voltage range between 0.8 V and up to 3.0 V for some materials.

Figure 6 shows the contrast between a reversibly charged electrochemical capacitor material (RuO₂) and an irreversibly chargeable battery-type material (Pb/PbCl₂).
 

Related article

Bibliography

• Power Limitations of Supercapacitor Operation Associated with Resistance and Capacitance Distribution in porous Electrode Devices, W. G. Pell and B. E. Conway, "Journal of Power Sources" Vol. 105, pp 169-181, 2002.

• Advances in Electrochemical Capacitors and Hybrid Power Systems, R. J. Brodd, D. H. Doughty, K. Naoi, M. Morita, C. Nanjundiah, J. H. Kim, and G. Nagasubramanian (editors), The Electrochemical Society Proceedings, Vol. 2002-7, Pennington, NJ, 2002.

• Bicentennial of Alessandro Volta's Invention of the "Electric Pile", B. E. Conway, "Canadian Chemical News" Vol. 52, No. 1, pp 15-17, January 2000.
  Available at: http://www.accn.ca/accn2000/january2000/pg12-22.pdf (the second article in the file).

• Electrochemical Supercapacitors: Scientific Fundamentals and Technological Applications, B. E. Conway, Kluwer Academic/Plenum Publishing, New York, 1999.

• The Role and Utilization of Pseudocapacitance for Energy Storage by Supercapacitors, B. E. Conway, V. Birss, and J. Wojtowicz, "Journal of Power Sources" Vol. 66, pp 1-14, 1997.

• Ruthenium Dioxide: a New Interesting Electrode Material, Solid State Structure and Electrochemical Behavior, S. Trasatti and G. Buzzanca, "Journal of Electroanalytical Chemistry" Vol. 29, pp A1-A5, 1971.

• Michael Faraday, a Biography, L. Pearce Williams, Chapman and Hall, London, 1965.

• On Porous Electrodes in Electrolyte Solutions, R. de Levie, "Electrochimica Acta" Vol. 8, pp 751-780, 1963.

• Low Voltage Electrolytic Capacitor, H. L. Becker, U.S. Patent 2,800,616, 1957.

• Equilibrium Properties of Electrified Interfaces, R. Parsons, in "Modern Aspects of Electrochemistry" Vol. 1, pp 103-179, J. O'M. Bockris (editor), Butterworths, London, 1954.

• The Electrical Double Layer and the Theory of Electrocapillarity, D. C. Grahame, "Chemical Reviews" Vol. 41, pp 441-501, 1947. Available on the WWW.

• Physical Chemistry, (Chapter 1) S. Glasstone, MacMillan, London, 1940.

• Leyden Jar, "Encyclopaedia Britannica" Vol. 13, p 989, 1929.

• Zur Theorie der Elektrolytischen Doppelschicht, O. Stern, �Zeitschrift f�r Elektrochemie and angewandte physikalische Chemie� Vol. 30, pp 508-516, 1924. Available on the WWW.

• The Electron in Chemistry, J. J. Thomson, Chapman and Hall, London, 1923.

• A Contribution to the Theory of Electrocapillarity, D. L. Chapman, �The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science� Ser. 6, Vol. 25, pp 475-481, 1913. Available on the WWW.

• Sur la constitution de la charge �lectrique � la surface d'un �lectrolyte, L. G. Gouy, �Journal de Physique Th�orique et Appliqu�e� Ser. 4, Vol. 9, pp 457-468, 1910. Available on the WWW.

• Sur la constitution de la charge �lectrique � la surface d'un �lectrolyte, L. G. Gouy, �Comptes rendus hebdomadaires des s�ances de l'Acad�mie des sciences, Paris� Vol. 149, pp 654-657, 1909. Available on the WWW.

• On the Charge of Electricity Carried by Gaseous Ions, J. J. Thomson, "Philosophical Magazine" Vol. 5, pp 346-355, 1903.

• Ueber einige Gesetze der Vertheilung elektrischer Str�me in k�rperlichen Leitern mit Anwendung auf die thierisch-elektrischen Versuche (in German), H. von Helmholtz, "Annalen der Physik und Chemie, Leipzig" Vol. 89, pp 211-233, 1853. Available on the WWW.

Listings of electrochemistry books, review chapters, proceedings volumes, and full text of some historical publications are also available in the Electrochemistry Science and Technology Information Resource (ESTIR). (http://knowledge.electrochem.org/estir/)

Return to: Top – Encyclopedia Home Page – Table of Contents – Author Index – Subject Index – Search – Dictionary – ESTIR Home Page – ECS Home Page